Change in Material Per Turn
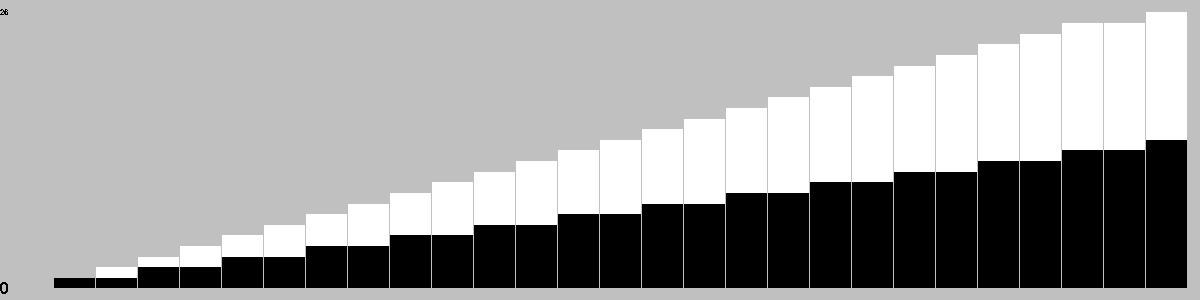
This chart is based on a single playout, and gives a feel for the change in material over the course of a game.
Connect your opposite sides orthogonally.
A crosscut is a 2x2 pattern of stones consisting of two diagonally adjacent black stones and two diagonally adjacent white stones. On your turn:
a) If there are no crosscuts on the board, you must place a stone of your colour on an empty point of the board such that it forms no crosscuts containing a stone that was placed by the opponent on their latest turn. If there is no such placement available, you must pass. Passing is otherwise not allowed.
b) If there are one or more crosscuts on the board, you must take a friendly stone from one of those crosscuts and place it on a different empty point where it doesn't reate any other crosscuts. If no such point exists, the stone is simply removed from the board.
General comments:
Play: Combinatorial
Family: Combinatorial 2016
Mechanism(s): Connection
Components: Board
| BGG Entry | Cation |
|---|---|
| BGG Rating | 6.2 |
| #Voters | 5 |
| SD | 3.18748 |
| BGG Weight | 0 |
| #Voters | 0 |
| Year | 2016 |
| User | Rating | Comment |
|---|---|---|
| luigi87 | 9 | This is quite possibly my best square board connection game. |
| russ | N/A | Another of Luis's clever interesting connection game, rather more dynamic than typical connection games: lots of stones get moved around due to the cross-cut rules. The cross-cut rules cause an effect which reminds me (favorably) of ko fights in Go, making a threat elsewhere so you can come back and play a crosscut or solidly connect to avoid a future cross-cut. The cross-cut rules are a bit weird to wrap your head around at first glance, but the sample game PDF in the files section is worth looking at and makes it all clear if there is any doubt. |
| mrraow | 6 | Finding new ways to break crosscuts on a square grid is all very well, but this feels akin to putting a sticking plaster on a missing limb. |
| milomilo122 | N/A | Incredibly cool concept. Cognitively demanding. Currently fairly opaque to me but it feels like this is clearing up slowly as I play more. |
| Kaffedrake | 5 | Square-grid Hex adaptation which handles diagonal connections differently from its sibling Rhode: by contesting such a connection you temporarily evict an opposing piece, so you don't lose tempo until you actually fill in the connection, nor are you required to follow through. I think we can all agree that cations are better than dogions, and Cation definitely feels more subtle than Rhode. They share the feature of being heavily dependent on proper pie rule execution for balance. |
| Smjj | 1 | |
| AndrePOR | N/A | Print & Play Edition |
| nuhuo1234 | 10 | |
| cdunc123 | N/A | This is a square grid connection game that I want to try. |
| AI | Strong Wins | Draws | Strong Losses | #Games | Strong Win% | p1 Win% | Game Length |
|---|---|---|---|---|---|---|---|
| Random | |||||||
| Grand Unified UCT(U1-T,rSel=s, secs=0.01) | 36 | 0 | 0 | 36 | 100.00 | 38.89 | 46.50 |
| Grand Unified UCT(U1-T,rSel=s, secs=0.03) | 36 | 0 | 3 | 39 | 92.31 | 58.97 | 42.13 |
| Grand Unified UCT(U1-T,rSel=s, secs=0.07) | 36 | 0 | 6 | 42 | 85.71 | 71.43 | 39.52 |
| Grand Unified UCT(U1-T,rSel=s, secs=0.20) | 36 | 0 | 12 | 48 | 75.00 | 64.58 | 38.98 |
| Grand Unified UCT(U1-T,rSel=s, secs=0.55) | 36 | 0 | 4 | 40 | 90.00 | 52.50 | 33.88 |
| Grand Unified UCT(U1-T,rSel=s, secs=1.48) | 36 | 0 | 9 | 45 | 80.00 | 46.67 | 33.04 |
Level of Play: Strong beats Weak 60% of the time (lower bound with 90% confidence).
Draw%, p1 win% and game length may give some indication of trends as AI strength increases; but be aware that the AI can introduce bias due to horizon effects, poor heuristics, etc.
| Size (bytes) | 31501 |
|---|---|
| Reference Size | 10577 |
| Ratio | 2.98 |
Ai Ai calculates the size of the implementation, and compares it to the Ai Ai implementation of the simplest possible game (which just fills the board). Note that this estimate may include some graphics and heuristics code as well as the game logic. See the wikipedia entry for more details.
| Playouts per second | 8852.89 (112.96µs/playout) |
|---|---|
| Reference Size | 626330.95 (1.60µs/playout) |
| Ratio (low is good) | 70.75 |
Tavener complexity: the heat generated by playing every possible instance of a game with a perfectly efficient programme. Since this is not possible to calculate, Ai Ai calculates the number of random playouts per second and compares it to the fastest non-trivial Ai Ai game (Connect 4). This ratio gives a practical indication of how complex the game is. Combine this with the computational state space, and you can get an idea of how strong the default (MCTS-based) AI will be.
| 1: Black win % | 55.79±2.55 | Includes draws = 50% |
|---|---|---|
| 2: White win % | 44.21±2.52 | Includes draws = 50% |
| Draw % | 0.00 | Percentage of games where all players draw. |
| Decisive % | 100.00 | Percentage of games with a single winner. |
| Samples | 1468 | Quantity of logged games played |
Note: that win/loss statistics may vary depending on thinking time (horizon effect, etc.), bad heuristics, bugs, and other factors, so should be taken with a pinch of salt. (Given perfect play, any game of pure skill will always end in the same result.)
Note: Ai Ai differentiates between states where all players draw or win or lose; this is mostly to support cooperative games.
Rotation (Half turn) lost each game as expected.
Reflection (X axis) lost each game as expected.
Reflection (Y axis) lost each game as expected.
Copy last move lost each game as expected.
Mirroring strategies attempt to copy the previous move. On first move, they will attempt to play in the centre. If neither of these are possible, they will pick a random move. Each entry represents a different form of copying; direct copy, reflection in either the X or Y axis, half-turn rotation.
| Game length | 38.74 | |
|---|---|---|
| Branching factor | 48.19 | |
| Complexity | 10^64.32 | Based on game length and branching factor |
| Computational Complexity | 10^7.06 | Sample quality (100 best): 25.57 |
| Samples | 1468 | Quantity of logged games played |
| Distinct actions | 4061 | Number of distinct moves (e.g. "e4") regardless of position in game tree |
|---|---|---|
| Good moves | 1339 | A good move is selected by the AI more than the average |
| Bad moves | 2722 | A bad move is selected by the AI less than the average |
| Terrible moves | 1665 | A terrible move is never selected by the AI Too many terrible moves to list. |
| Samples | 1468 | Quantity of logged games played |

This chart is based on a single playout, and gives a feel for the change in material over the course of a game.
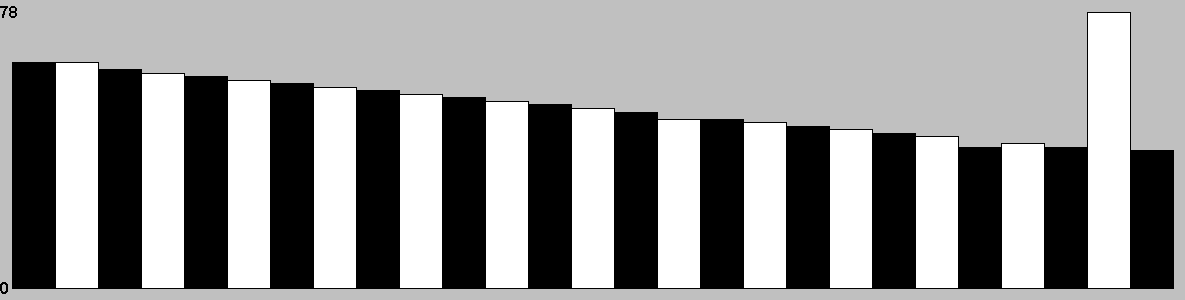
Table: branching factor per turn.
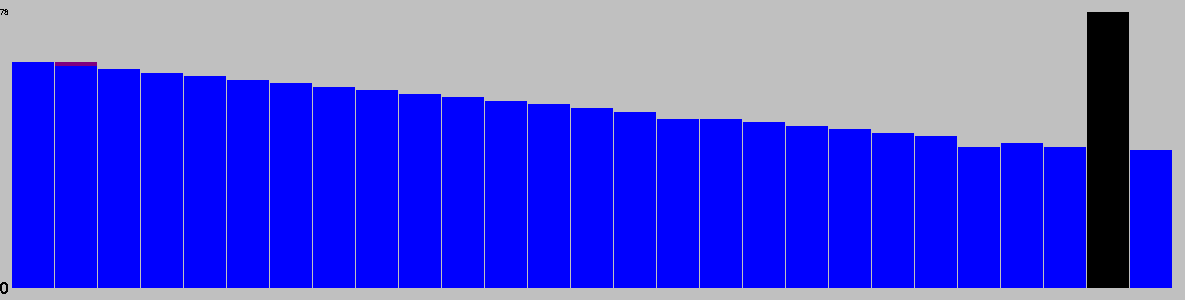
This chart is based on a single playout, and gives a feel for the types of moves available over the course of a game.
Red: removal, Black: move, Blue: Add, Grey: pass, Purple: swap sides, Brown: other.
| 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| 1 | 64 | 4160 | 87488 | 3347598 |
Note: most games do not take board rotation and reflection into consideration.
Multi-part turns could be treated as the same or different depth depending on the implementation.
Counts to depth N include all moves reachable at lower depths.
Inaccuracies may also exist due to hash collisions, but Ai Ai uses 64-bit hashes so these will be a very small fraction of a percentage point.
No solutions found to depth 4.
| Moves | Animation |
|---|---|
| d2,Swap,e2 |  |
| g2,Swap,d6 |  |
| g2,Swap,g6 |  |
| c3,Swap,d2 |  |
| c3,Swap,d4 |  |
| c3,Swap,f5 |  |
| e3,Swap,d5 |  |
| f3,Swap,c3 |  |
| f3,Swap,f4 |  |
| f3,Swap,f5 |  |
| f3,Swap,g6 |  |
| g3,Swap,f6 |  |
| Puzzle | Solution |
|---|---|
 White to win in 3 moves |
Selection criteria: first move must be unique, and not forced to avoid losing. Beyond that, Puzzles will be rated by the product of [total move]/[best moves] at each step, and the best puzzles selected.